[ad_1]
The 1982 SAT infamously held a math concern so tricky that even its creators didn’t involve a accurate solution. The botch required the rescoring of 300,000 tests, scholastic victims of the knotty coin rotation paradox.
Here’s how the paradox functions: Spot two quarters flat on a desk so that they are touching. Keeping 1 coin stationary on the desk, roll the other quarter all over it, retaining edge get in touch with amongst the two devoid of slipping. When the moving quarter returns to its starting off place, how several full rotations has it designed? In other terms, how lots of occasions has George Washington returned to his upright posture in the graphic underneath? If you dig puzzles like this, get a moment to consider about it.
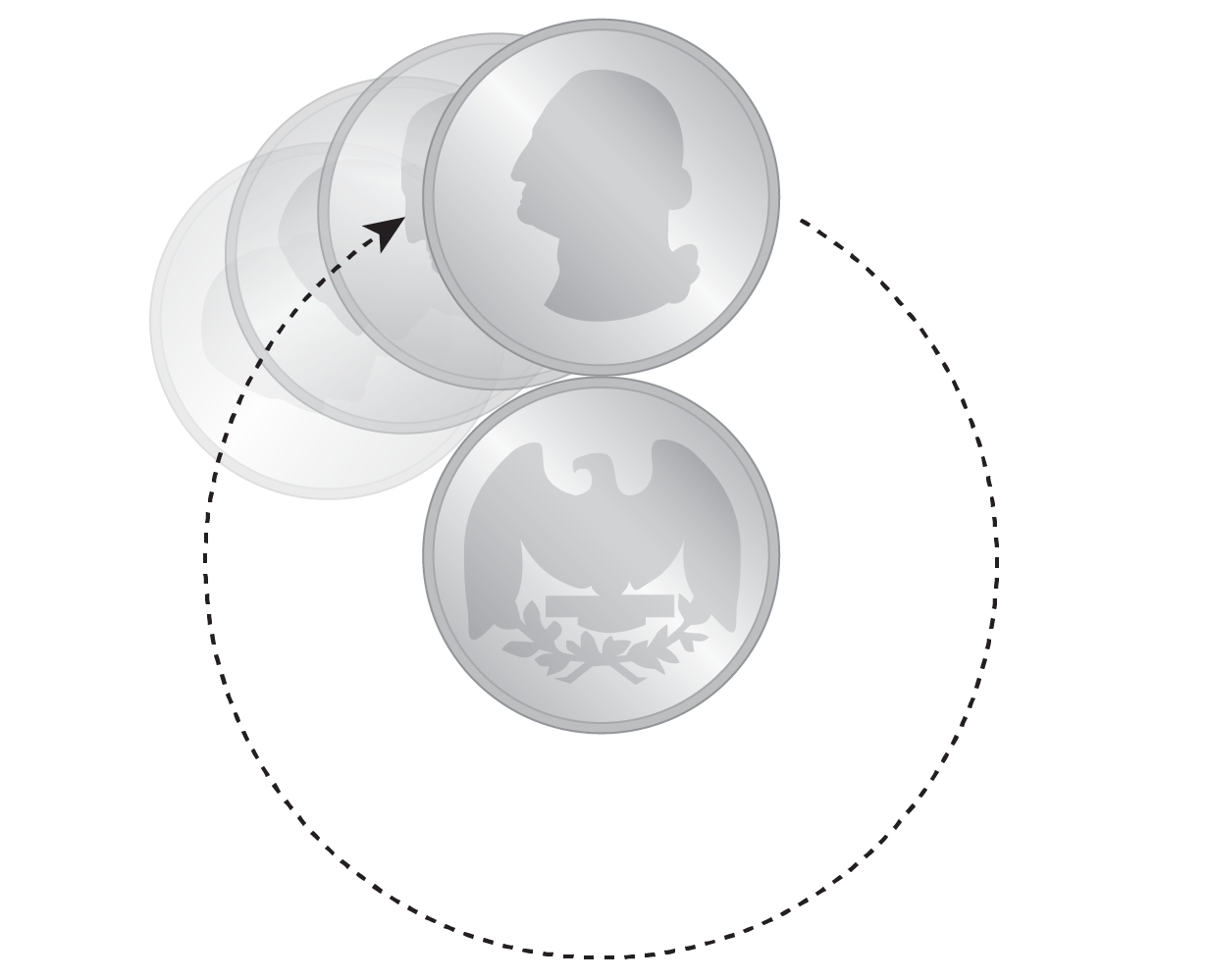


A lot of men and women suspect that George will make just one comprehensive rotation. A quarter’s circumference is about 3 inches all around. So the moving quarter rolls together a route with a duration of three inches, the exact distance as its individual circumference. If we wrap a string all around a quarter and roll it along a three-inch path, unfurling the string as we go, then certainly a few inches of string will unfurl—just enough for a solitary rotation.
In simple fact, the shifting quarter would make specifically two entire rotations by the time it returns to its primary place. The phenomenon defies typical perception. If you come across it tricky to settle for, I motivate you to examination it for yourself. Any two disks of equivalent dimensions will do.
Linked: Why the ‘Sleeping Attractiveness Problem’ Is Keeping Mathematicians Awake
The variation of this trouble that snuck its way onto the 1982 SAT math segment had a small variation: the middle disk was larger sized than the just one rolling all over it. Here’s a version of that issue, with the wording a little modified for clarity:
The radius of circle B is a few moments the radius of circle A. Beginning from the placement proven in the determine, circle A rolls all-around circle B. When circle A returns to its commencing issue, how many rotations will it have completed?
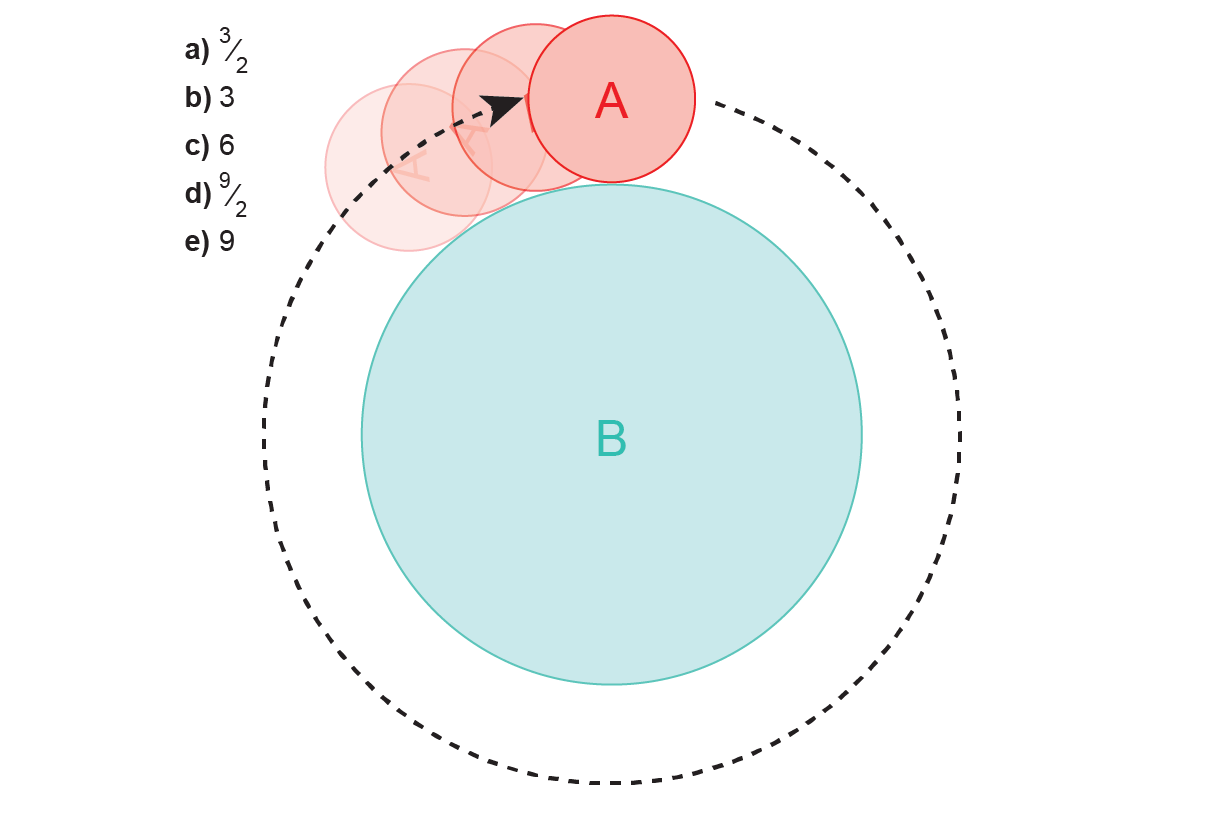


Does that appear to be familiar? In this article, we’re explained to the much larger circle’s radius is a few instances that of the smaller circle. This indicates the very same for the circumferences of the two circles: B is 3 times more time all around its perimeter than A. It is tempting to reason that the smaller circle could “unwrap” itself particularly three occasions to encase the greater 1. So “3” was the intended many-decision solution on the SAT. In fact, circle A will make four rotations on its trip—again, just just one extra rotation than intuition expects. The paradox was so much from the check writers’ awareness that 4 wasn’t made available as an possibility between the achievable solutions, so even the most astute college students were pressured to post a mistaken response. A few of the 300,000 college students who took the take a look at made up of the concern noted the issue to the School Board, and each individual exam experienced to be rescored.
So why is there an more rotation? The technique that led us astray earlier mentioned does comprise some wisdom. Rolling a quarter together a three-inch straight-line route would include only 1 rotation. Also, a modest circle rolling in a straight line with a duration that is three occasions its diameter would rotate three periods. So the circular condition of the route by some means triggers a rotation of its personal. To see why, envision rolling a quarter close to the perimeter of a little poppy seed. George will rotate after even even though the duration all over the seed is negligible. So there are two resources of rotation: one from rolling along a route (the lengthier it is, the much more rotations) and a further from revolving around an item, which contributes one rotation irrespective of its sizing.
One more valuable standpoint will come from imagining rolling a quarter all-around a sq.. Each edge of the sq. is a straight-line segment, and George’s head will spin after for each individual a few inches of duration, but the minute you achieve a corner, the quarter will have to rotate farther to apparent that corner. (All over again, attempt this for your self if it’s difficult to photograph.) It turns out that this excess rotation at the corners is specifically 90 degrees, which success in 1 total rotation (360 levels) by the time the coin traverses all four corners of the sq. and returns to its start out. Likewise, rolling about a triangle would entail 120-degree rotations close to every single corner.
The impact scales up to celestial bodies. The moon famously has a dim side and generally reveals the same encounter to us Earthlings. Quite a few individuals erroneously interpret the unchanging see of the moon to signify that it must not spin about its axis like Earth does. If the moon didn’t spin throughout its orbit, although, we would see its darkish aspect from some places on Earth. You can show this with your individual fists—hold 1 regular and orbit the other all-around it, without the need of any rotation. Observers standing on one of your stationary knuckles will get a diverse glimpse of the orbiting fist at distinctive occasions. To eternally cover its derriere, the moon has to rotate after each and every time it completes an orbit. (This ideal parity concerning orbit time and rotation time is not an astronomical coincidence but relatively an illustration of a phenomenon called tidal locking. We’re also sidelining our relativistic reference frame, with apologies to Albert Einstein.) With the coins and the SAT dilemma, we noticed that there had been two sources of rotation: some from “straight-line” rolling along any route and 1 more from revolving about an item. The moon doesn’t do any straight-line rolling. If the Earth ended up flat, the moon would glide higher than it without the need of rolling. So the one rotation of the moon is entirely due to the fact of its revolution about the extremely spherical Earth.
If you had an aerial watch of the solar process, how numerous instances would you see Earth finish a rotation in a standard 12 months? A lot of would say 365, but however yet again, they would slide just one shorter of the accurate response: 366. (Be aware this has almost nothing to do with leap many years, which are a totally individual make a difference.) Humanity has outlined a working day to be the amount of time it requires for the sunlight to return to the identical locale in the sky. It is convenient to normally have the sunshine immediately overhead at noon. But when Earth completes one rotation, the sunshine actually hasn’t quite returned to its perch in the sky however. Let us revisit the SAT diagram to see what’s likely on—only this time we’ll mark a mounted dot on the little circle and notice what happens to the dot as that circle rolls about the large 1:
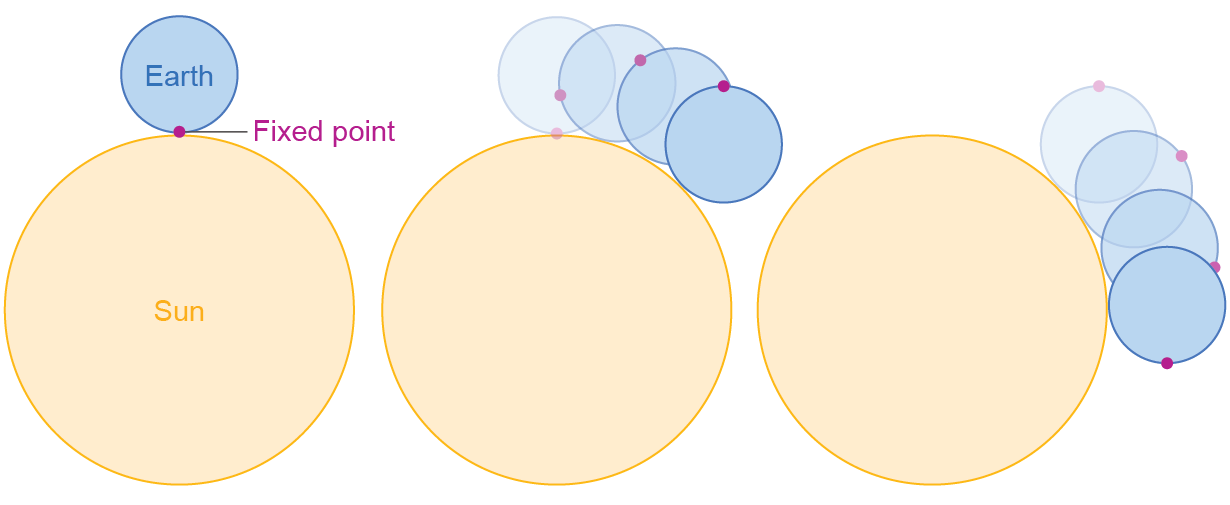


Imagine of the massive circle as the sunlight, the little circle as Earth (not to scale) and the dot as a fastened level on our planet. In the initial panel, the dot stares immediately at the sun. It is particularly midday. In the very last panel, the little circle has done a total rotation (the dot points down all over again), but notice that it is not noon for a person standing at the dot. The little circle would need to have to edge ahead a minor further than a single rotation for the dot to kiss the major circle once more. Also, though Earth completes a rotation in 23 several hours and 56 minutes (which is termed a sidereal working day), it takes 4 extra minutes for the sun to return to its overhead area in the sky, yielding our definition of a 24-hour working day. In excess of the study course of 365 times, these four added minutes of rotation per day increase up to a person additional rotation.
Thanks for rolling along with us on this tour of cash, tests mistakes and planetary motion—it’s sufficient to make anyone’s head spin.
[ad_2]
Source url



