[ad_1]

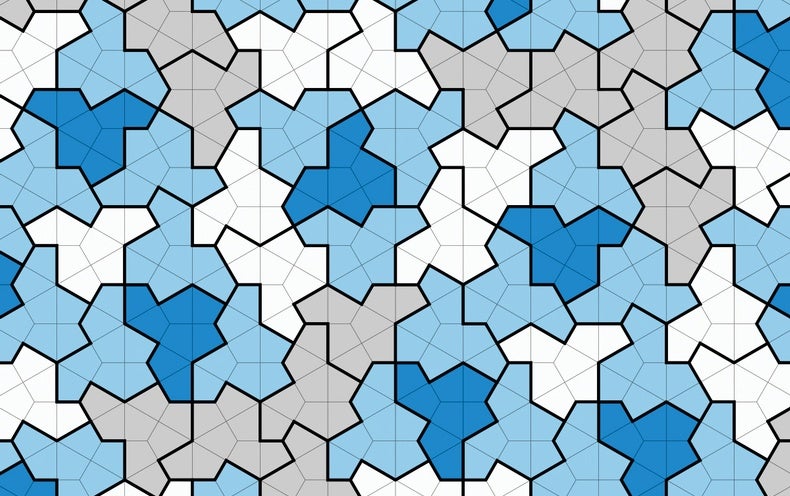
David Smith, a math hobbyist in Yorkshire, England, has found out a 13-sided form that eluded mathematicians for a long time. The craggy, hatlike form is termed an “einstein,” based mostly on the German for “one stone.” If you made use of einstein-shaped tiles to address your rest room floor—or any flat surface area, even if infinitely large—they would in shape together properly but never ever type a repeating sample. For decades mathematicians have been hunting for tile shapes like these that can kind only nonrepeating preparations, termed aperiodic tilings. They started off with sets of several unique tiles: the to start with established, identified in 1964, essential 20,426 unique tiles, which was later simplified to 103. By 1974 mathematician Roger Penrose had located two tile styles that, when combined in a mosaic, never ever shaped a repeating sample.
But was it probable to form an aperiodic tiling with tiles of only a person shape—the hypothetical einstein? Doris Schattschneider, a retired mathematician affiliated with Moravian University with know-how in tessellations, had been skeptical about the probability of a genuine einstein at any time remaining learned. “That’s why it was so startling that not only was this found, but it can be these types of a easy tile,” she states. “To me, it can be a total anomaly.” The tile Smith uncovered in November 2022, although he was experimenting with different styles applying a software package identified as PolyForm Puzzle Solver, was astonishing in its class. Produced up of proper-angled kites, it was almost nothing like the gnarly, difficult form of condition lots of mathematicians would have predicted.
Smith e-mailed Craig Kaplan, a pc scientist at the University of Waterloo in Ontario, who acknowledged the shape’s likely. Although the mosaic it established seemed not to have a repeating sample, the duo needed to mathematically establish it never ever would—even if the mosaic had been infinitely significant. They enlisted software program developer Joseph Samuel Myers and University of Arkansas mathematician Chaim Goodman-Strauss, who experienced equally labored with tiling and combinatorics in the earlier.
The researchers applied two procedures to prove they had a genuine einstein on their palms. Initially, they confirmed that the hatlike tiles, when organized alongside one another, shaped four certain kinds of styles. Including a lot more tiles varieties even greater variations of those people similar styles, or “supertiles” the additional tiles additional, the greater the supertiles come to be. Mathematicians have proved that this hierarchical framework indicates the tiling cannot be break up into repeating sections and therefore have to be aperiodic. For the 2nd proof, the group invented a new strategy that could assess the hat tilings with the nonperiodic tilings of far better-recognised shapes known as polyiamonds. In the course of action, the crew also showed that one could make an infinite number of very similar einsteins by tweaking some of the sides’ lengths.
Even with these proofs, mathematicians have not however formulated a broader theory for what makes this uncomplicated hat condition so particular. “This is even now extremely mysterious,” says mathematician Rachel Greenfeld of the Institute for Innovative Examine in Princeton, N.J. There may well be far more classes of einstein tiles out there, waiting around to be uncovered, but the geometric hat is not revealing any clues, claims Marjorie Senechal, a retired mathematician affiliated with Smith University: “These items you stumble on.”
Editor’s Note: A earlier variation of this tale appeared on the net with the title “Newfound Mathematical ‘Einstein’ Form Produces a Never ever-Repeating Sample.”
[ad_2]
Source connection